Angular Momentum Conservation

UNDER CONSTRUCTION

Description of the Experiment

This control room allows the confirmation of angular momentum conservation by colliding a spinning disk with another. Moreover, the disk inertia momentum can be extrapolated from energy conservation principles.

Experimental Apparatus

The experimental apparatus is based in a PC hard-disk-drive motor and its spinning disk. A second disk is held on top of it and can be dropped by a servo motor actuator.

The apparatus´ motor can be used as a generator equipped with a switchable resistor acting as an electromagnetic brake. The braking current&voltage characteristic is measured allowing a rigorous energy dissipation calculation.

Protocol - Angular Momentum Conservation

Figura 1: Angular velocity (rpm) as a function of time for a collision at 1000 rpm.

A disc with a total mass of 115g are accelerated by the hard-drive motor until they reach a selected angular velocity. In this instant the motor is disconnected from supply and the discs allowed to rotate freely being their speed monitored. When a certain pre-determined speed is reached the servo lets the suspended discs with a total mass of 69g initially at rest fall on top of the rotating disc.

Data taken from the experiment is given and plotted with the disc speed in function of time.

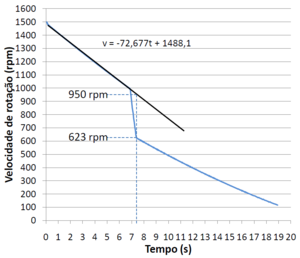
Figure1 is a plot created in Microsoft Excel using the table of results of an experience in which the servo let's the suspended discs fall when the discs below reach 1000 rpm.

Doing a linear regression between the deceleration and fall of the discs, it's possible to obtain the predicted rotational speed at the time that the falling discs stop sliding over the bottom discs.

Physics

Using the following quantities:

L - angular momentum

I - moment of inertia

ω - angular velocity

m - mass in rotation.

We have for the angular momentum conservation:

\[L_i=L_f\] \[I_i \omega_i=I_f \omega_f\] \[\frac{I_i}{I_f}=\frac{\omega_f}{\omega_i}\] \[\frac{\frac{m_i\left (r_1^2+r_2^2 \right )}{2}}{\frac{m_f\left (r_1^2+r_2^2 \right )}{2}}=\frac{\omega_f}{\omega_i}\] \[\frac{m_i}{m_f}=\frac{\omega_f}{\omega_i}\]

The experimental results give:

\[\frac{\omega_f}{\omega_i}=\frac{623}{950}=0,656\]

while the predicted mass ratio is

\[\frac{m_i}{m_f}=\frac{115}{115+69}=0,625\]

Evaluating the accuracy

\[\frac{\left|0,656-0,625\right|}{\left|0,625\right|}\times 100=4,9\%\]

The speed ratio is different from the mass ratio by 4,9% which confirms the angular momentum conservation.

Advanced Protocol - Moment of Inertia Evaluation

Figura2: rotational speed

Figura3: voltage across one of the resistors

Figura4: compensated voltage

A disc with a total mass of 115g are accelerated by the hard-drive motor until they reach a pre-defined angular velocity. In this moment the motor is disconnected and the disc allowed to rotate freely. When a certain speed that the user defines previously is reached, a relay puts each motor winding in parallel with a resistor which resistance is the same as the motor's windings. These resistors will dissipate energy acting as an electromagnetic brake. This energy can be accounted and using the table values provided (disc speed and voltage across the resistors)in function of time.

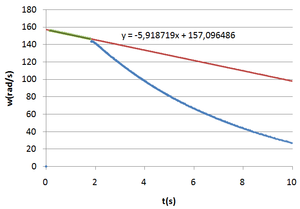
Figure2 and 3 are plots created in Microsoft Excel using the table of results of an experience in which the relay turns on when the rotating discs reach 1000 rpm.

The difference in the deceleration slope is clear. At first the discs are slowed only by the kinetic friction but when the relay turns on the resistors and motor windings are put in parallel and energy starts being dissipated through the resistors. The contribution of the resistors to the deceleration gives a changing in the slope observed and the disc energy can be evaluated by the difference between the slopes.

Quantifying:

\[\frac{d\omega_{res}}{dt}=\frac{d\omega_{atrito+res}}{dt}-\frac{d\omega_{atrito}}{dt}=-194,860-(-79,234)=-115,626rpm/s = -115,626\times\frac{2\pi}{60}=-12,11rad/s^2\]

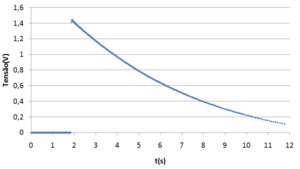
The kinetic friction must also be taken into account in the voltages so that the energy dissipated by the resistors corresponds to the energy loss given by the difference between slopes. This can be done adding the function adjusted to the first data series to the second and adjusting a new function.

To clarify, a voltage of 0,0241(t-6,42)V will be added to each point in the table of results after the time at which the relay turns on and a new function is adjusted to that data series. The result of this process is shown in Figure4.

Using the voltage given by the adjust function in Figure4 and knowing that the resistance is 5,3Ω in each of the 3 resistors, the dissipated power is calculated by:

\[P=VI=V\frac{V}{R}=\frac{V^2}{R}\]

The energy dissipated in the 3 resistors is obtained integrating the dissipated power over time:

\[\int_{6,67}^{9,62}\frac{dE}{dt}dt=3\int_{6,67}^{9,62}\frac{V^2}{R}dt=0,313J\]

The energy of a solid body in rotation and it's derivative is given by:

\[E=\frac{I\omega^2}{2}\;\;\;\;\;\;\;\;\;\;\frac{dE}{dt}=I\omega'\omega\]

The last equation is integrated knowing that \(\int_{6,67}^{9,62}\frac{dE}{dt}dt=-0,313J\) (the "-" is there because the energy is dissipated), ω' is constant = -12,11rad/s^2 (comes from the difference between slopes) and ω is given by the deceleration adjust function in rad/s,

\[\int_{6,67}^{9,62}\frac{dE}{dt}dt=\int_{6,67}^{9,62}I\omega'\omega \; dt\] \[-0,313=I(-12,11)\int_{6,67}^{9,62}\omega \; dt\] \[\frac{-0,313}{-12,11}=I\int_{6,67}^{9,62}-20,41t+230,93 dt\] \[I=\frac{-0,313}{-12,11\times 190,837}\] \[I=1,354\times 10^{-4}kg\, m^2\]

Now, the discs in this experience are in fact rings of interior and exterior radius 13mm and 47mm respectively. Their moment of inertia is then:

\[I=\frac{m\left(r_1^2+r_2^2\right)}{2}=\frac{0,115\left(0,013^2+0,047^2\right)}{2}=1,367\times 10^{-4}kg \; m^2\]

Evaluating the accuracy,

\[\frac{\left|1,354\times 10^{-4}-1,367\times 10^{-4}\right|}{\left|1,367\times 10^{-4}\right|}\times 100=0,9\%\]

The experimental results of this experience differ only 0,9% from the theoretical expectations!