Difference between revisions of "Angular Momentum Conservation"
| Line 9: | Line 9: | ||
The aparatus also has a group of braking resistors that can be connected in parallel with the motor windings. This setup allows the realization of the second protocol, centered in the study of the moment of inertia. | The aparatus also has a group of braking resistors that can be connected in parallel with the motor windings. This setup allows the realization of the second protocol, centered in the study of the moment of inertia. | ||
| − | =Protocol1 - Angular | + | =Protocol1 - Conservation of Angular= |
[[File:Discos_velocidade_protocolo1.png|thumb|alt=|Figura1: velocidade de rotação]] | [[File:Discos_velocidade_protocolo1.png|thumb|alt=|Figura1: velocidade de rotação]] | ||
| Line 31: | Line 31: | ||
m - mass in rotation. | m - mass in rotation. | ||
| − | <div style="text-align: center;">We have for the angular momentum | + | <div style="text-align: center;">We have for the conservation of angular momentum:</div> |
\[L_i=L_f\] | \[L_i=L_f\] | ||
\[I_i \omega_i=I_f \omega_f\] | \[I_i \omega_i=I_f \omega_f\] | ||
| Line 38: | Line 38: | ||
\[\frac{m_i}{m_f}=\frac{\omega_f}{\omega_i}\] | \[\frac{m_i}{m_f}=\frac{\omega_f}{\omega_i}\] | ||
| − | <div style="text-align: center;"> | + | <div style="text-align: center;">The experimental results give:</div> |
\[\frac{\omega_f}{\omega_i}=\frac{623}{950}=0,656\] | \[\frac{\omega_f}{\omega_i}=\frac{623}{950}=0,656\] | ||
| − | <div style="text-align: center;"> | + | <div style="text-align: center;">while the predicted mass ratio is</div> |
\[\frac{m_i}{m_f}=\frac{115}{115+69}=0,625\] | \[\frac{m_i}{m_f}=\frac{115}{115+69}=0,625\] | ||
| − | <div style="text-align: center;"> | + | <div style="text-align: center;">Evaluating the accuracy</div> |
\[\frac{\left|0,656-0,625\right|}{\left|0,625\right|}\times 100=4,9\%\] | \[\frac{\left|0,656-0,625\right|}{\left|0,625\right|}\times 100=4,9\%\] | ||
| − | + | The velocity ratio is different from the mass ratio by 4,9% which confirms the conservation of angular momentum. | |
Revision as of 18:44, 13 October 2012
UNDER CONSTRUCTION
Description of the Experiment
This control room allows the confirmation of angular momentum conservation and the measuring of the moment of inertia of rotating discs.
Experimental Apparatus
The experimental apparatus is based in a PC hard-drive to which were added a servo and a group of discs that the servo keeps above the rotating discs. This setup allows the realization of the first protocol, centered in the study of angular momentum conservation.
The aparatus also has a group of braking resistors that can be connected in parallel with the motor windings. This setup allows the realization of the second protocol, centered in the study of the moment of inertia.
Protocol1 - Conservation of Angular
5 discs with a total mass of 115g are acelerated by the hard-drive motor until they reach 1500rpm. In this moment the motor is disconnected, the discs rotate freely and their velocity is read. When a certain velocity that the user defines previously is reached, the servo let's 3 suspended discs with a total mass of 69g initially at rest fall on top of the rotating discs.
In the end of the session a table is provided with the disc velocity function of time, whose values will be used in a plot created in some program the user chooses.
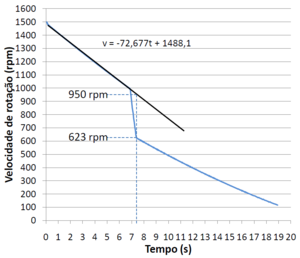
Figure1 it's a plot created in Microsoft Excel using the table of results of an experience in which the servo let's the suspended discs fall when the discs below reach 1000 rpm.
Doing a linear regression between the deceleration and fall of the discs, it's possible to obtain the predicted velocity of the discs at the time that the falling discs stop sliding over the bottom discs.
Using the following quantities:
L - angular momentum
I - moment of inertia
ω - angular velocity
m - mass in rotation.
\[L_i=L_f\] \[I_i \omega_i=I_f \omega_f\] \[\frac{I_i}{I_f}=\frac{\omega_f}{\omega_i}\] \[\frac{\frac{m_i\left (r_1^2+r_2^2 \right )}{2}}{\frac{m_f\left (r_1^2+r_2^2 \right )}{2}}=\frac{\omega_f}{\omega_i}\] \[\frac{m_i}{m_f}=\frac{\omega_f}{\omega_i}\]
\[\frac{\omega_f}{\omega_i}=\frac{623}{950}=0,656\]
\[\frac{m_i}{m_f}=\frac{115}{115+69}=0,625\]
\[\frac{\left|0,656-0,625\right|}{\left|0,625\right|}\times 100=4,9\%\]
The velocity ratio is different from the mass ratio by 4,9% which confirms the conservation of angular momentum.