Quantum interpretation of three polarizers

If we conduct the "Polaroide" experiment by doing a scan, lets say, of 180º rotation, we will conclude that the photons probability should be proportional to a sinusoidal function, like a [math] sin^2(\theta)[/math]. The sinus has to be squared as any probability can't be negative. Note that [math] sin^2(\theta)[/math] is still a sinusoidal function.

Let's describe the polarization state of light as a two-dimensional vector, as illustrated in the figure. Vertically polarized light corresponds to a vector pointing upwards (0, 1), horizontally corresponds to (1,0). We use Dirac notation to represent these vectors, |V> and |H> respectively. An arbitrary vector is written as \(|α〉=cos⁡α |V〉+sin⁡α |H〉 \).

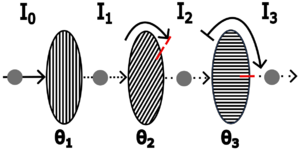
Quantum mechanics explains how to calculate: 1) the probability of transmission of these states through a polarizer, 2) the state at the exit of the polarizer. When the state \(|V>\) passes through the second polarizer, oriented at 45°, we have that: The transmission probability is given by

[math] 〖Prob=|〈V|P_(45°) |V〉|〗^2=1/2 [/math]

Expected drift in frequency when plasma is generated inside the chamber.

Expected drift in frequency when plasma is generated inside the chamber.

Expected drift in frequency when plasma is generated inside the chamber.

Expected drift in frequency when plasma is generated inside the chamber.